My Unit Plan: Math 8 Surface Area + Volume

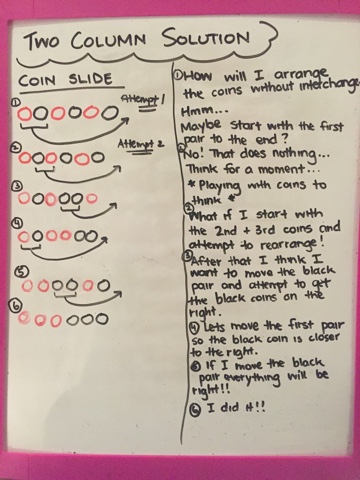
https://drive.google.com/file/d/0B_JvSjJRS_QQOXVuWW0ydmEzbkU/view?usp=sharing

Here is the link to my Unit Plan!

Reflection: John Mason

1) Do Mason's ideas might connect with inquiry-based learning in secondary school mathematics? (And why or why not?)

2) How might Mason's ideas about questions in math class be incorporated into your unit planning for your long practicum?

1 )

Mason's ideas definitely align with inquiry learning in secondary math. Inquiry allows teachers to not only be able to question their students but also allow their students to question them in a thought-provoking way. Mason's definition of a teacher who exemplifies good questioning is very similar to an inquiry-based teacher, who focuses on question-based learning. These teachers are able to create students who can ask thoughtful questions and be able to answer thoughtful questions by thinking critically.

2 )

A teacher is able to outline questions for his or her students in order to be able to incorporate questioning into their lessons. They may be able to note down possible questions they think their students may benefit from them asking. They can also note down possible questions their students may have, and outline paths they can create in order to allow them to get there. Following the path of John Mason, this would be an amazing addition to a unit plan.

Reflection: Dave Hewitt

In his classroom, Dave Hewitt made it imperative to his students to have a proper wait time. He did many lessons with his students and asked questions, but always gave an appropriate wait time, in a way that kept his students engaged in the lesson. He also found it very important to repeat his key points in order to ensure his students were understanding and absorbing every word he was saying. He made his lessons more so a conversation than a lecture, by incorporating the ideas of every student rather than simply transmitting knowledge. This way students had a memory of what they had learned rather than just notes on a paper. Finally, the most important part of Hewitt's lessons were when he would encourage his class to say the answer to his questions in unison. It allowed students to gauge their understanding while getting help from their peers along the way.
Of all of Dave Hewitt's classroom techniques, I definitely find the technique of his class saying answers in unison to be the most useful. While I can see how they would all be useful in their own ways, this is the one I would use the most. It is a clear way of seeing if the students understand, and a later reaction time, or no response from students can show that a student may not exactly understand the concept. I really enjoyed this video and hope to see more from Dave Hewitt in the future.

Micro-Teaching: Reflection

For my group's micro-teaching, we did a lesson on Graphing Relations from Math 10. We altogether felt the lesson went well but could have been better. Our point was to incorporate some sort of inquiry and technology into the lesson in order to keep our students engaged and still have them learn something. While I feel that we did succeed in getting our topic through to the class, one thing that we definitely could have worked on was the Kahoot quiz. It was evident that without the proper labelling of the axes, students were left unsure of what the answer to each question was for sure. The intention was to allow them room to decide what they thought in terms of the axes, but in reality all we did was confuse them. In the future, I will definitely do my best to make sure something like this does not happen again.

Micro-Teaching: Peer-Evaluations

Two Column Solution: Coin Slide Puzzle

Micro-Teaching: Lesson Plan

Topic: Graphing Relations
Grade: 10
Partner: Sissi

Objective/Goals: Students will be able to describe a possible situation for a given graph and sketch a possible graph for a given situation.

Time: 15 minutes

Strategies to help learn: Class is set in groups to allow students to work together. Also, it is inquiry based learning; students will develop critical thinking and collaborative skills

Hook: Kahoot quiz serves as a hook

Materials required: Computer, colorful envelopes (group activity), large paper for the group activity, markers

Assessment: The group activity will act as formative assessment of their knowledge, and the kahoot will serve as a check of prior knowledge.

Assumed prior knowledge: It is assumed that students will know the axes of a graph, as well as the coordinates of a graph, and be able to plot a graph given the points

Development of idea/skill:
** Possibly start off by checking for prior knowledge on rate of change (go over constant, not constant, and no rate of change using desmos?)

• Start off with Kahoot Quiz
• state that students are able to get into groups (MAX: 2) so this allows students to collaborate
• Group Activity: groups receive envelope and either have to create a situation for a given graph, or create a graph for a given situation
• if time permits, each group gives their answer
• Conclusion/come together and talk about underlying message of lesson

Conclusion: Go over key points of the lesson (i.e. rate of change)

Further extensions/Applications:  Extra envelopes for students who finish earlier, talk about speed in physics (driving and the speed you drive)

• exponential growth can be related to decay and half-life, and also finances (analyzing stock market history…?)

Article Reflection: Arbitrary And Necessary

Article Reflection

1) What does Hewitt mean by "arbitrary' and "necessary"? How do you decide, for a particular lesson, what is arbitrary and what necessary?

In his article, Hewitt describes the terms "arbitrary" and "necessary". By arbitrary, he means this is something that a student learns and is told to memorize. There aren't good explanations for these things that would make sense to the student so they are always told to memorize. Necessary refers to something that a student would be able to figure out on their own. These are things they could teach themselves with the proper "awareness" - proper background in order to understand and answer these questions.
In order to decide what is arbitrary and necessary, it is important to note what you intend for the students to know and learn by the end of the lesson. Once you do this, you can split the points into either arbitrary and necessary based on whether a student would be able to figure it out on their own through "awareness", or whether they would just need to be taught it and told to memorize it.

2) How might this idea influence how you plan your lessons, and particularly, how you decide "Who does the math" in your math class?

This idea is a major component that can be considered before creating a lesson plan. It is important to understand which parts of your lesson will be merely "memorized", and which parts will force the students to think for themselves and figure out the questions on their own based on the background they have been taught. When the teacher is deciding which aspects are necessary, those will entail students "doing the math", whereas arbitrary aspects will just entail memorization. 

Reflecting on SNAP Math fair

I really enjoyed the SNAP math fair, and all the work that the students put into it really seemed to have paid off! They were clearly very excited to have visitors at their stations to explain their problems and the reason behind choosing the problem.
One problem I particularly liked was this one about collecting all of the totem poles in the most correct way without doubling over your steps. Not only was the problem tricky but still solvable, the students who presented their problem had excellent presentation skills and were very engaged the entire duration of our visit to the station! I was particularly impressed by this station but still had a very good experience at every other station.
After viewing the SNAP math fair, and seeing exactly how it works, I truly feel like this could be implemented in my practicum high school.

SNAP Math Fair Booklet Reflection

Question

Could you, and would you, run a SNAP Math Fair in your practicum high school? Why/ Why not? If you can imagine doing so, how would you adapt the Math Fair to your school and classes, and why?

If I was to run a SNAP math fair at my practicum school, I feel that my students would be very receptive and willing to take part. Of course not all students would be willing to take part, but I know for the most part there would be a healthy interest. I would definitely love to do something like this as I feel that most students would take interest and want to participate. In order to have a successful fair, a huge part of it is student interest. 

I would create a general group of grade 8 students who were interested, and gear the questions to suit students of all levels in order to fit any student who was interested. There would be no competitive aspect to encourage students to participate knowing there would be no consequences if their project was not adequate enough in someone else's eyes. Instead of making the fair open to all students, making it open to only grade 8 students makes it easier to organize, and possibly even keeping it to my practicum class of 8's makes it even easier. Students would be able to choose their own question they feel passionate about - they could possibly even call it a "passion project". They could then put their "projects" or math questions on display for a class of grade 7 students, who could come in and view their work. 

Reflection: Battleground Schools

For this blog response, please comment on the fraught history of mathematics education in North America and the ways that you think this might affect your own situation as a math teacher.

I really enjoyed reading this article as it touches on many points that teachers have to deal with throughout their careers. It is interesting to see how much the change of curriculum can affect many teachers. It is also interesting how black and white math education was in the past; there was no room for expression in mathematics, and there was only room for material to be transmitted from teacher to student. It is not surprising at all that students who were not going into post-secondary studies in mathematics were not thought of during the process of putting together a curriculum standard. As teachers, we always unconsciously dream all of our students will grow to go down the same path as we did. However, this is completely not the case. Students choose the past that is best for them and that should be okay.

As a future teacher, it would definitely affect my teaching if the curriculum was to change. Looking at the new curriculum, teaching is now becoming much different than it was before. Being a student of the old curriculum, I can somewhat sympathize with the teachers discussed in the article who were not comfortable with the new curriculum standard; however, as teachers, it is our job to be comfortable enough with the material to relay it to our students. As long as the standards consider all students and not just those going to post-secondary, it is important that teachers do their best to become comfortable with the new standard so their students can also be comfortable. 

Teachable subject: Braiding hair

I really enjoyed the whole process of the teachable subjects in our groups! I thought it was amazing how we went from knowing nothing of each topic to every basic element!
My teachable was on braiding hair. The people in my group thought my lesson was overall good, but said I required a better hook. I feel that if I incorporated a better hook the overall flow of my lesson would have been much better. I also noticed most of my group mentioned I lacked organization in my lesson, so this is something I will need to work on for the future.
Overall, I really enjoyed presenting my lesson to my group and am excited for future attempts.

Soup Can Puzzle

Volume = pi x r^2 x h
Goal: To find out radius and height of the real water tank in order to figure out the volume
Photo -
Height of bike: 5.5 cm
Diameter water tank: 10.5 cm

Real Life -
Campbells soup: 11.5 cm x 8.5 cm
Height of bike: 110 cm (looking up the height of a regular bike)

Using the information above, my goal is to find the diameter and height of the water tank.
I found the diameter by finding the ratio of the height of bike and water tank in the picture vs real life:
10.5 cm : 5.5 cm = d : 110, so the diameter is d = 210 cm.

Using the information above, I then found out the projected height of the water tank. I used the diameter and height of the soup can, and the projected diameter of the real water tank in order to find a projected height of the water tank:
210 cm : h = 8.5 cm : 11.5 cm, where h = 284.12 cm.

Therefore, the volume of the soup can water tank would be
V = pi x r^2 x h
= pi x (105)^2 x (284.12) = 9840797.08 cm^3 = 9840.79708 Litres

Lesson Plan: Braiding Hair

Objective/Goals: Students will be able to braid their own hair, or another's hair.
Time: 10 minutes

Strategies to help learn: students will be able to 

Hook: Long hair can be very beautiful but very hard to manage. Today I am going to show you how many men and women with longer hair manage their hair by the process of braiding.

Materials required: ribbon, paper, tape

Assessment: Students will show understanding from makeshift "hairs" (ribbon).

Assumed prior knowledge: Some students will know how to braid already, and some will not. 

Development of idea/skill: Students will be taught how to begin by parting hair into 3 pieces, followed by bringing alternating sides to the middle.
e.g. Beginning with the right piece, bring over the middle piece into the middle, and bring the middle to that side. Follow the same pattern continuing with the left side and then the right.

Conclusion: Today we learned how to braid hair, and I hope everyone has gained 

Further extensions/Applications: Some further extensions to what we learned today are the fishtail and french braid.  

Letters from Students!

Letter One:
Dear Ms. B,
I just wanted to write this letter to thank you for all the hard work you put into teaching our class. You made exactly enough time for every student, and even though it was tough, you made sure everyone in the class felt important. There was never a point in the class when students didn't understand because you went through all the material meticulously. I always felt engaged in the classroom and felt excited to learn. I really appreciate all of the times you kept your door open in order to let us students come in and ask questions before and after school.
I just wrote to tell you thanks!
Sincerely,
Bill

Reflection:
I've always wanted to become a teacher for the sole reason that I want to make a difference in the lives of students. It has always been my goal to make sure I have as much time as possible for my students in order to make sure they are understanding everything being taught. While I know this is a dream, I want to do my best to make it a reality.

Letter Two:
Dear Ms. B,
I am writing to let you know that I had a lot of problems with the way you taught me. You constantly pressed the class about attending before and after school sessions, even though no one was ever interested in coming. I felt you were not personable with your teaching, and didn't make it apply to anything we would ever use in our lives. I constantly battled with myself over if I even liked math in high school because I was so bored in your class. I don't mean this to be harsh, but just wanted to let you know so you could improve for the future.
Sincerely,
Bill

Reflection:
This letter is based on fears I have as a teacher. I always go back and forth about whether I even want to do before and after school sessions for the reason that I feel most students would not want to attend. I also worry that students will not like my approach to learning and shut down and end up not liking math in the future. In my eyes, all it takes is one bad teacher to turn a student away from a subject, and my ultimate concern is becoming that teacher for a student.

Hyperboloid Structure: Reflection

The project my group did on our hyperboloid structure was one of the most memorable presentations I will have done during this program, and this I know for sure. I knew immediately I wanted to do the hyperboloid structure from the beginning, since it was the one that stuck out most to me.

Building the structure was inspiring as it did not take us long to figure out our own method of creating it. It was very easy to find the materials as well, as the skewers were from a market and the rubber bands were from a beauty supply store! Instead of the method shown online using paper to hold the skewers, we attached pairs of the skewers together and added more and more to the attached sets. We worked on two different models in order to find out which model would work better. Doing this allowed us to see faults in one model and see the strengths in the other. It was a great chance to work as a team, as me and Jacob worked on one model, while Etienne and Deeya worked on the other. Instead of two people, or one person, working on one model, we did something so everyone could work together!

The math art project we did has large correlation to conics – taught in secondary mathematics. When teaching this subject, it may be helpful for students to create the structure in order to better understand conics like hyperboloids and paraboloids. It would be a great way to teach and to learn the subject. Students could do extensions of their own in order to gain further understanding as well.

Furthermore, I feel that students of many ages can gain something from an experience of building our structure. It is simple enough that younger children can build it, as well as older students in later years of secondary school.

Dishes puzzle

I began my problem by writing out the constraints I had to work with:

- 2 people shared 1 dish of rice,

- 3 people shared 1 dish of broth,

- 4 people shared 1 dish of meat

I then noticed that in order to find out the number of people, I could see this relation:

1 dish/2 people + 1 dish/3 people + 1 dish/4 people = 6 dishes + 4 dishes + 3 dishes/ 12 people 

(by finding the LCM)

which would lead me to an answer of 13 dishes/12 people.

Since we know that there are 65 dishes, 

we can see that if we divide the number of dishes by the 13 dishes/12 people relation, we can cancel the "dishes" and get a result containing only the number of people.

Doing this,

65 dishes ÷ 13 dishes/12 people =  5 65 dishes x 12 people/13 dishes = 60 people

Therefore, without using algebra, you can see that there are a total of 60 guests.

I don't think cultural context has any effect at all because the problem would be just as doable for any student if there was no underlying cultural context. I think it is a good problem to have, but I do not think there is any strong, underlying cultural effect left.

Article Reflection: Math that Matters

Questions:

•Is mathematics 'neutral', or is it connected with social/ environmental justice?
•What are your ideas about the author's intentions in writing this textbook?
•Can these ideas from middle school math inspire teaching ideas for your secondary math classes?
•Are there topics in mathematics that are more or less possible to connect with social justice issues?

Reflection:

I feel that math is definitely driven by social and environmental change and justice, or it can be. It is ultimately up to the educator to decide whether to touch on outside factors related to math, or to just teach the basics. The intention of the author, in my eyes, was to open up the eyes of teachers in order to show them that there is more to teaching math than meets the eye. There is a definite way of incorporating the outside world to math. David Stocker's purpose was to give teachers the option of being able to bring social awareness into their teaching; this is wonderful as I feel that most teachers maybe feel confused about how exactly to incorporate these things into teaching, but Stocker gives great examples along with his writing. 

In secondary math classes, it can definitely be harder to incorporate social justice into teaching, but again, it is up to the teacher to attempt to do so. Personally, thinking of senior level math classes, I feel it would be more difficult to associate lessons with social justice, as students would just want to focus on the concepts in order to get a good mark for post-secondary. A teacher could try to connect probability with social justice issues, but topics like logarithms and transformations may a bit harder to relate to real-world problems, though this does not mean they are impossible to relate. 

Pro-D-Day Plans: BCAMT Conference

I will be attending the BCAMT conference in Whistler!

Reflection: Memory

In secondary school, math was my absolutely favourite subject. I had my teacher, Mr. B, to thank for that. He was that teacher that everyone had a healthy fear for, but was also the teacher that everyone wanted to impress. Through his thick exterior, he was generous to all his students, and always made sure everyone that needed extra attention got it. Though I never needed extra attention, he always made sure those of us doing better in the classroom were given the opportunity to be challenged. It was because of this teacher that I had such an amazing experience with secondary mathematics.

I never particularly had a terrible math teacher, but I did have a teacher who was very cut and dry about math. I was not challenged at all in the classroom, and did not feel my needs were met. I feel that it would have better if I was challenged and given more work to do, and I do not feel that I would been as bored if I had been given the opportunity to do more challenging activities in the classroom.

Reflection: TPI Survey

I felt the TPI survey matched my own personality very well. From the results above from the TPI survey, I am seen to be mostly nurturing, but still concerned with student development. My views on social reform are not particularly strong, which explains it being my lowest score.
I am opinionated on all of the sections the survey tested for, so I was surprised I did not have a higher score for all of the others as well. Overall, I understood I was mostly nurturing because I tend to care a lot for my students well-being in the classroom, all while making sure they know how to succeed academically.
In the future, I do want to work on being more even across the board when it comes to these topics. I feel they are all important aspects of teaching, so keeping them all in mind will allow for the success of the teacher. I don't think I'll ever lower my level of nurture, as I feel an extremely important part of teaching is being a caring - but still professional - individual that students can always look up to. I look forward to seeing how these aspects come through in my teaching.

Entrance Slip - How many squares in a chessboard ?

When thinking of how many squares are in a chessboard, I begin by thinking of a regular 8x8 chessboard.

In order to see all of the different types of squares that can be formed from this board, I must begin by thinking of the smallest squares that can be found, and work my way up to an 8x8 square.

To begin, I think of a 1x1 chessboard, seeing that it just has 1 square.

Moving to a 2x2 chessboard, one would see 5 squares.

* There is one large 2x2 square, and 4 1x1 squares.

In a 3x3 chessboard, one would see 14 squares

* There are 9 1x1 squares, 4 2x2 squares, and 1 3x3 square.

From these you can see that a pattern emerges:

For a 1x1 chessboard, 1^2 =1

For a 2x2 chessboard, 1^2 + 2^2 = 5

For a 3x3 chessboard, 1^2 + 2^2 + 3^2 = 14

etc.

When applying this pattern to an 8x8 chessboard, we see that 1^2 + 2^2 + 3^2 + 4^2 + 5^2 + 6^2 + 7^2 + 8^2 = 1+4+9+16+25+36+49+64=204.

From this we would see that there are 204 squares in a chessboard.

Initially, I entered this problem thinking of an obvious answer of 64 squares. I quickly realized there was more to this problem than I thought. I needed to find a way of calculating the number of squares in a quicker way than just counting. I began with the smallest size of squares and worked my way up to 3, before finding a pattern. I realized that through finding out the each number of squares, they were all "perfect squares", meaning they have a square root. Knowing perfect squares helps me, as well as deductive reasoning and seeing a pattern emerging. This problem could be extended by asking for the number of squares of a larger chessboard and checking understanding. 

Reflection

After discussing the differences and similarities of instrumental and relational learning, I still stand strong on my point of relational understanding. However, I do feel that instrumental learning has good points to it as well. Time is an important factor to making instrumental a strong point, while long term success allows relational understanding to come out on top.
My view is that both understandings should be combined in order to have a successful classroom. Students should be able to either learn the background before going into the step by step process of learning, but also be able to go the other way around. Teaching this way allows for a variety of different students to have their needs met.

Skemp Article Response

I had a very strong viewpoint in regards to the issue as I began this article, but as I read I began to question the views I was so certain of. The description of relational and instrumental understanding was raw and original, and I had never thought of teaching math as having two different paths. It became clear there was a divide between teachers who were only able to allow instrumental understanding, and teachers who only allowed relational understanding. I did not think there would be anything to attract me to allow students to understand instrumentally, until I came across reasons why it may be helpful, which pleasantly surprised me. Skemp's example of being in a new town also surprised me, as I had never seen a more thoughtful representation of relational and instrumental understanding.
My perspective on this issue is that teachers should always do their absolute best to relay relational understanding to their students, but it may not work for all students. Some students may take to learning theory very well, but some students may get confused and even pull away. A crucial part of being a good educator is being diverse with all students in the classroom; this can be achieved by attempting relational understanding with stronger students and instrumental understanding for students who may need more confidence with math. Once confidence has been built, attempts to change their understanding can be made.

Hello world! Welcome to my blog